
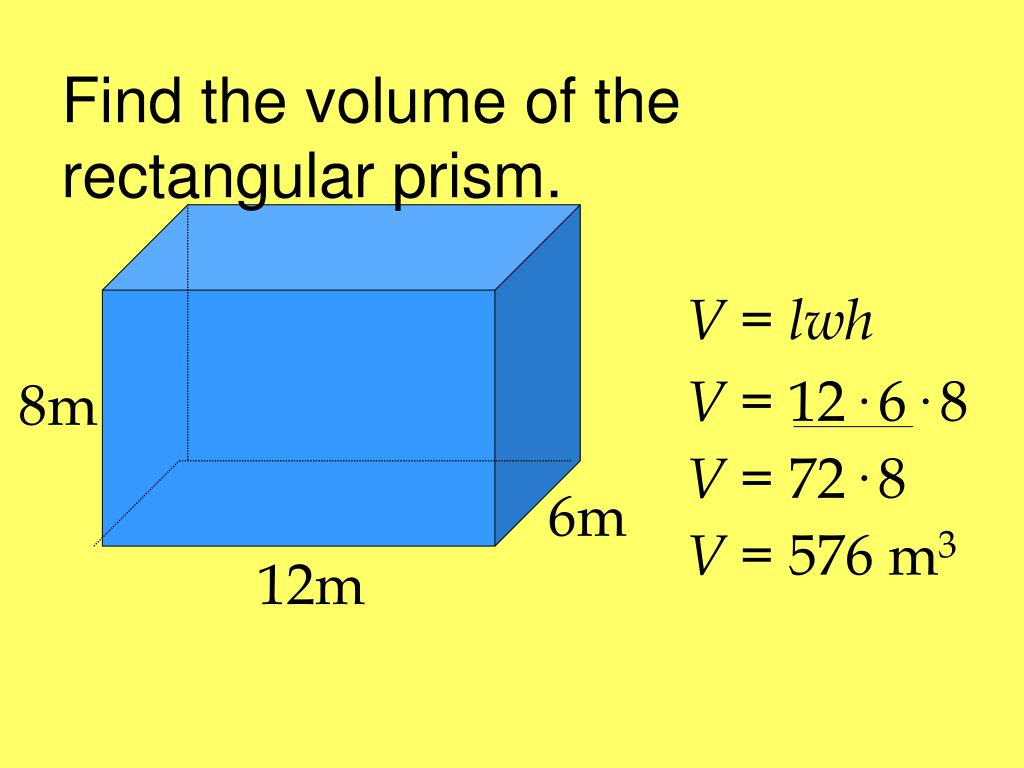
By designating one dimension as the rectangular prism's depth or height, the multiplication of the other two gives us the surface area which then needs to be multiplied by the depth / height to get the volume.
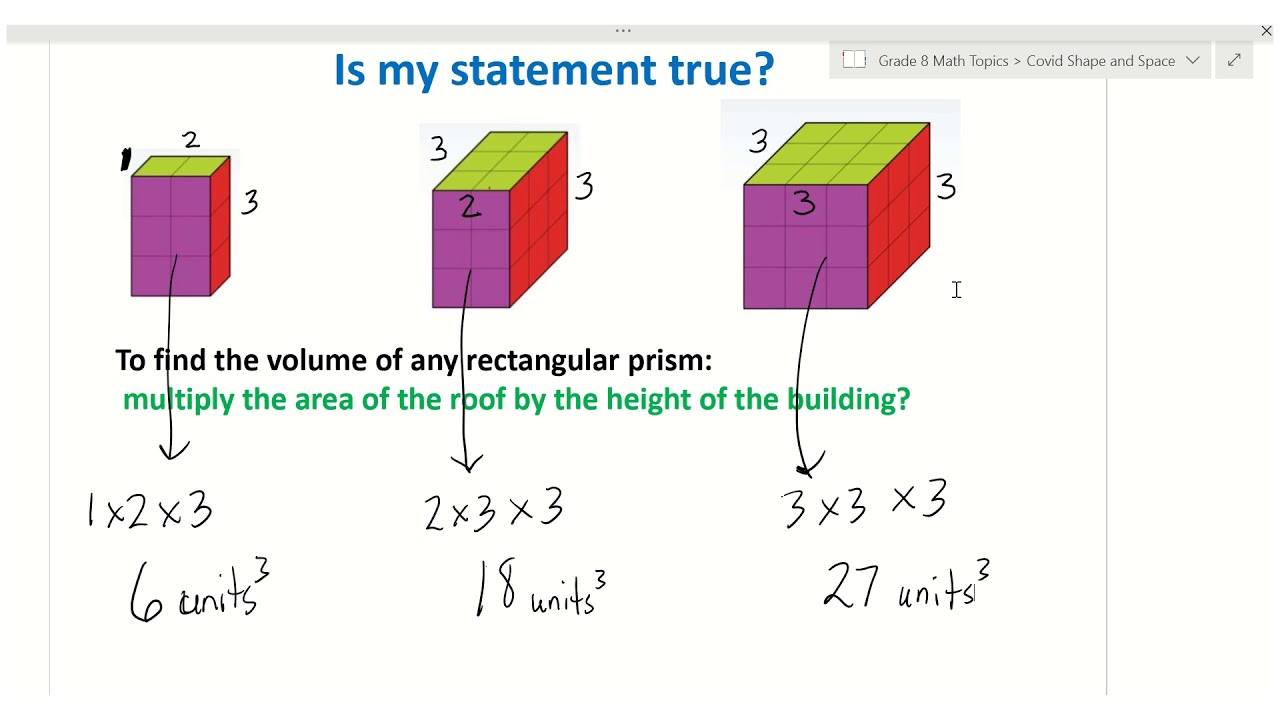
They are usually easy to measure due to the regularity of the shape. To calculate the volume of a box or rectangular tank you need three dimensions: width, length, and height. To find the volume of a rectangular box use the formula height x width x length, as seen in the figure below: For this type of figure one barely needs a calculator to do the math. It is the same as multiplying the surface area of one side by the depth of the cube. The only required information is the side, then you take its cube and you have found the cube's volume. The volume formula for a cube is side 3, as seen in the figure below: air conditioning calculations), swimming pool management, and more. Volume calculations are useful in a lot of sciences, in construction work and planning, in cargo shipping, in climate control (e.g. The result is always in cubic units: cubic centimeters, cubic inches, cubic meters, cubic feet, cubic yards, etc. All measures need to be in the same unit.
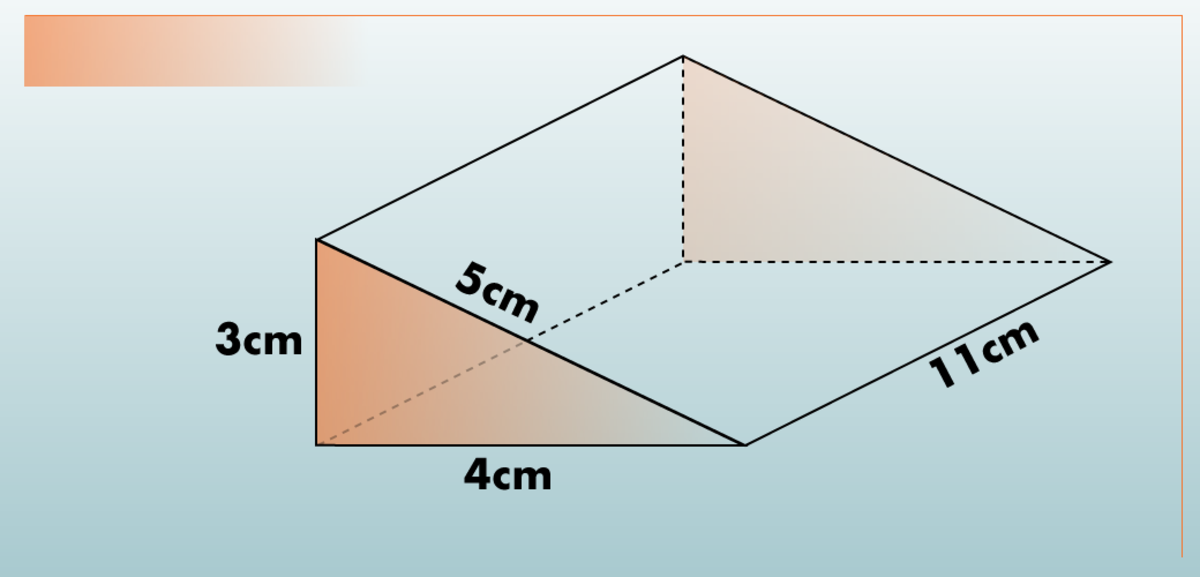
Below are volume formulas for the most common types of geometric bodies - all of which are supported by our online volume calculator above.


 0 kommentar(er)
0 kommentar(er)
